Office: 2844 East Hall
Phone (Cell): (734)-255-8610
Phone (Office): (734)-764-6897
Mail (Work):
David E Speyer
Department of Mathematics
2844 East Hall
530 Church Street
Ann Arbor, MI
48109-1043 USA
|
E-mail:
speyer@umich.edu Office: 2844 East Hall Phone (Cell): (734)-255-8610 Phone (Office): (734)-764-6897 Mail (Work): David E Speyer |
|
|
I am a profesor in the department of mathematics at the Univeristy of Michigan. I am married, and have three kids.
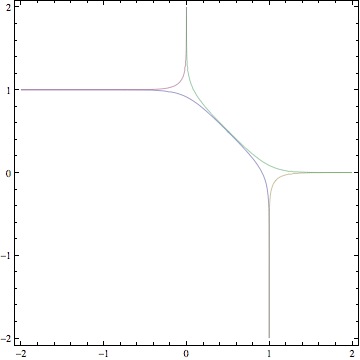
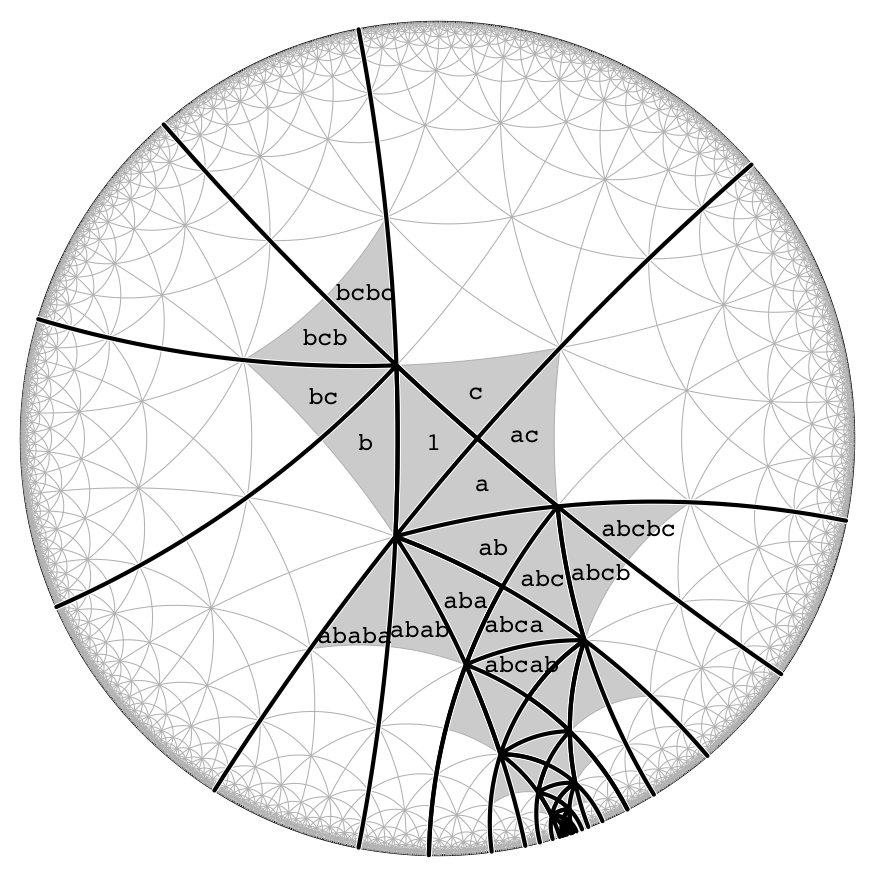
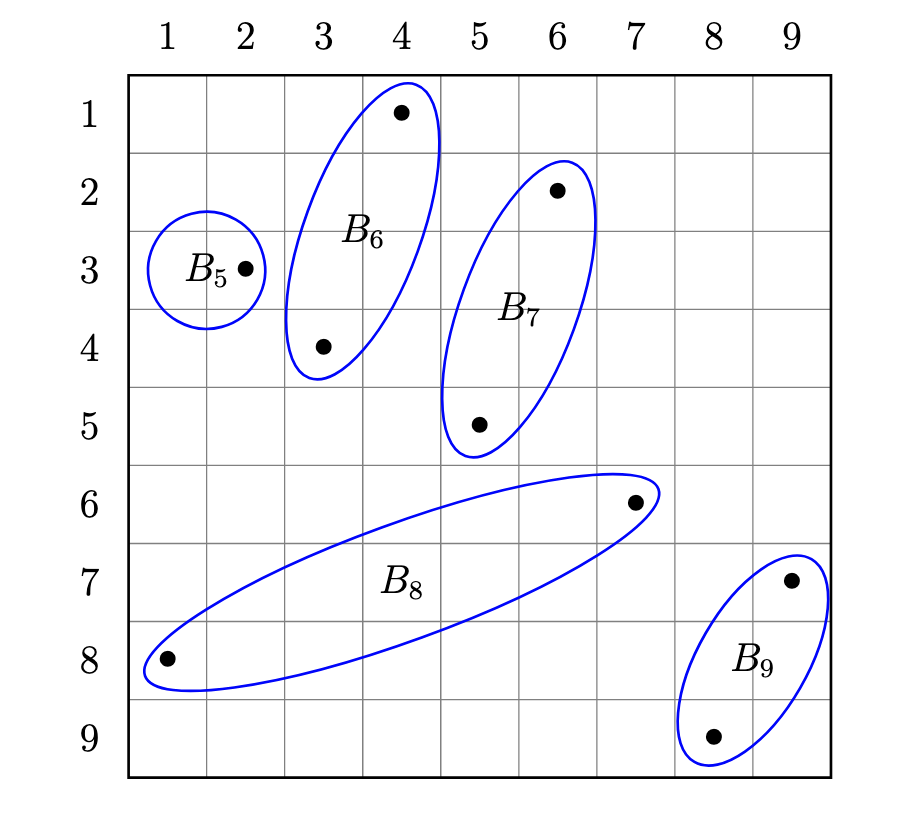
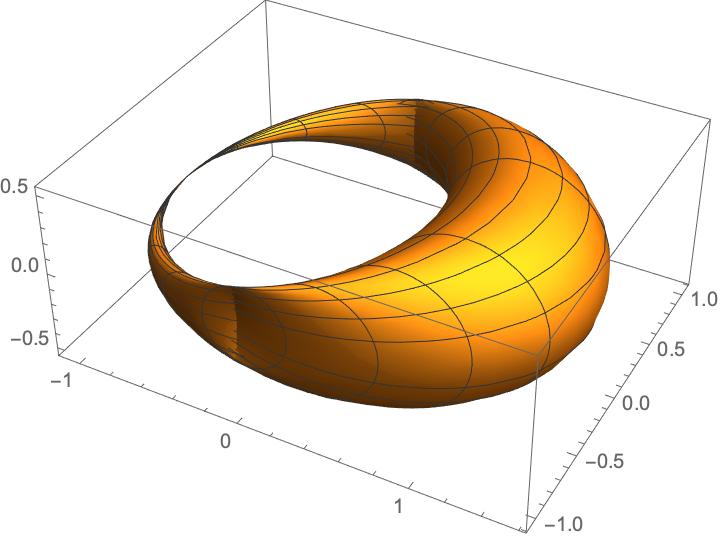
I enjoy algebraic problems with a combinatorial flavor. If you started out with a well motivated algebraic question but wound up with lots of little complicated pictures, I probably want to hear about it. Some topics I can usually be relied upon to think about: tropical geometry, cluster algebras, flag manifolds and other geometry of Lie Groups, interesting degenerations of algebraic structures, exact results and asymptopics of perfect matchings (eg Arctic Circle phenomena). I also know a reasonable amount of Number Theory and enjoy talking about it, although as yet this is not a research interest.
In Fall 2019, I am teaching Graduate Algebra I (Math 593) and Combinatorics of Coxeter Groups (Math 665).
You can see my past teaching here.
My current students are Gracie Ingermanson (cluster algebras, Bruhat cells) and Harry Richman (something with Tropical geometry and Weierstrass points). My graduated students are Nic Ford (Knutson's shifting construction for positroids), Giwan Kim (standard monomial theory and toric degenerations), Jake Levinson (higher dimensional analogues of the Mukhin-Tarasov-Varchenko theorem and K-theory of Grassmannians), Rohini Ramadas (Hurwitz spaces and connections to dynamics) and John Wiltshire-Gordon (representation stability, configuration spaces and representations of categories). .
I have supervised undergraduate research projects by Grant Barkley, Benjamin Branman, Andrew Gitlin, Patrick Lenning, David Rush, Shubhankar Sahai, Jonathan Schneider, Julio Cesar Soldevilla.
Before coming to the University of Michigan as a professor, I was a Clay Research Fellow. In that capacity, I spent two years (2005-2007) at the University of Michigan and three (2007-2010) at MIT.
I have a Ph. D. in mathematics from UC Berkeley, where I was a student of Bernd Sturmfels. My thesis is on tropical geometry, an approach to turning algebraic geometry problems into polyhedral geometry. Before coming to Berkeley, I was an undergraduate at Harvard. While there, I worked for Jim Propp's research group REACH and wrote an undergraduate thesis on the Eichler-Shimura correspondence under William Stein. I spent the rest of my time doing theater tech, hanging out with science fiction fans and working on Les Phys — the physics musical!
I spent four years as a counselor at PROMYS, a number theory program for high school students, and highly endorse it either as a place to study or to work. I spent my own high school summers at MOP, which I found great but works better for some people than for others. I went to High School at Choate Rosemary Hall and to Middle School at Talcott Mountain Academy. If you are a young nerd in Connecticut, looking for a middle school or after school program, I highly recommend Talcott.
